|
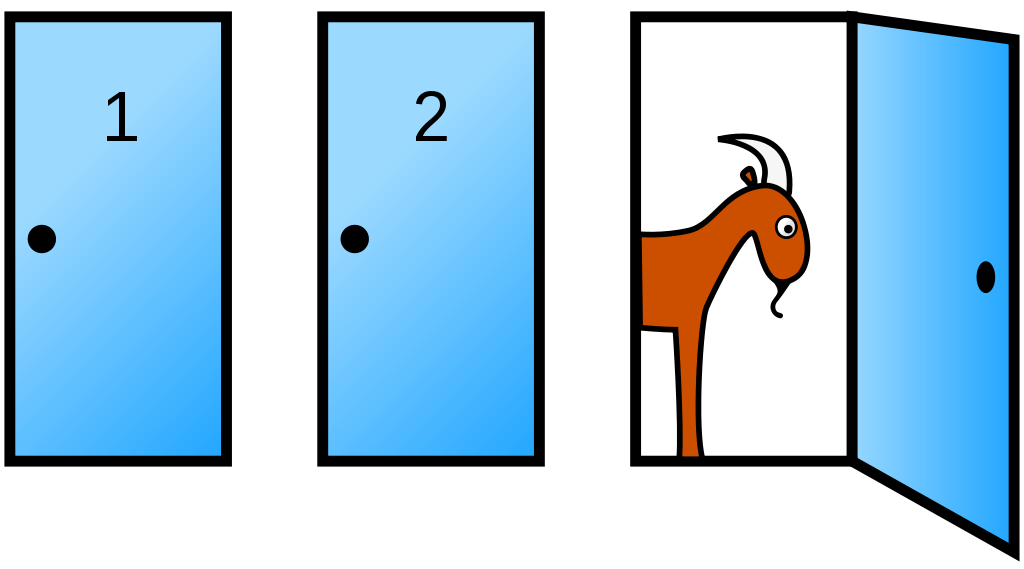
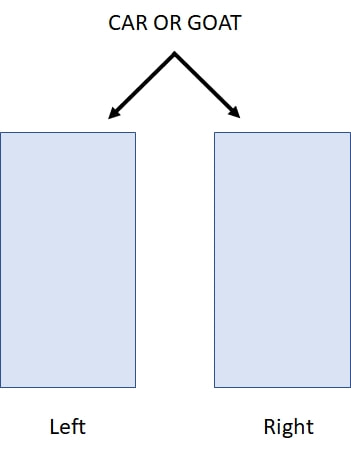
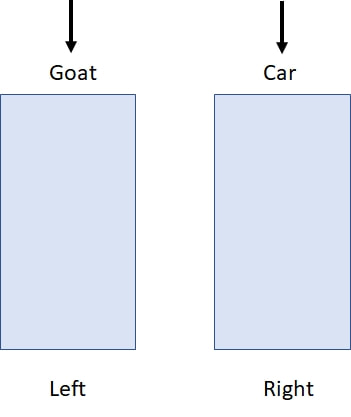
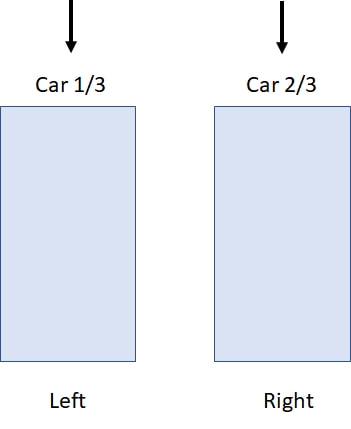
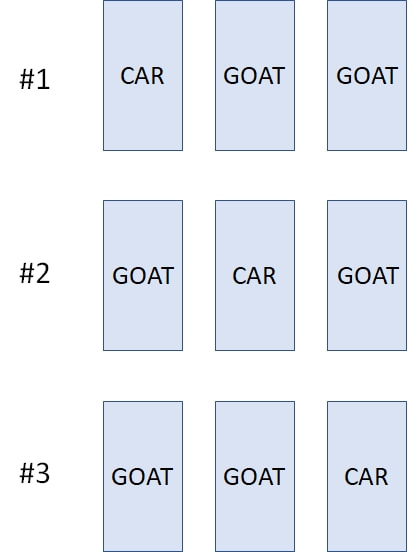
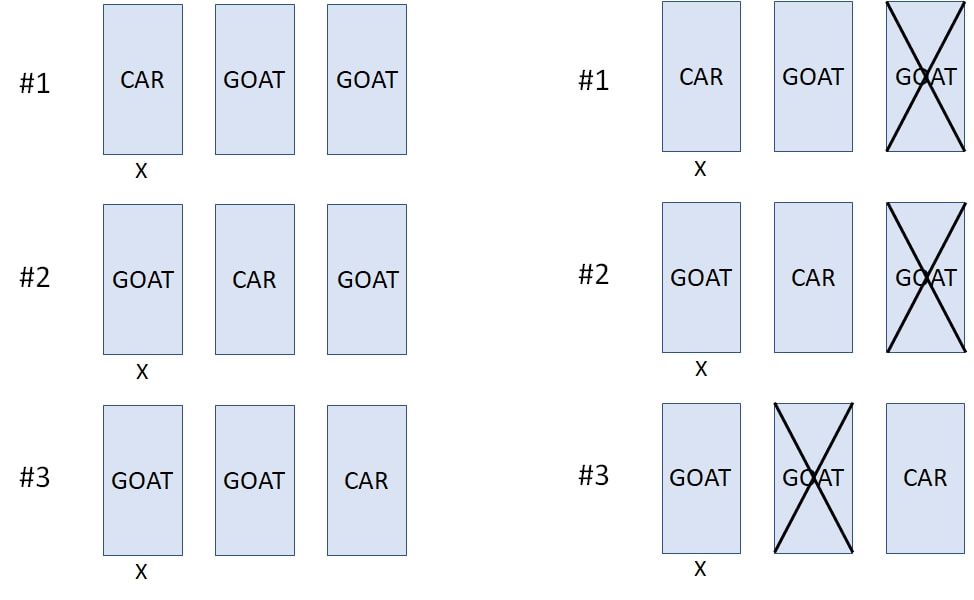
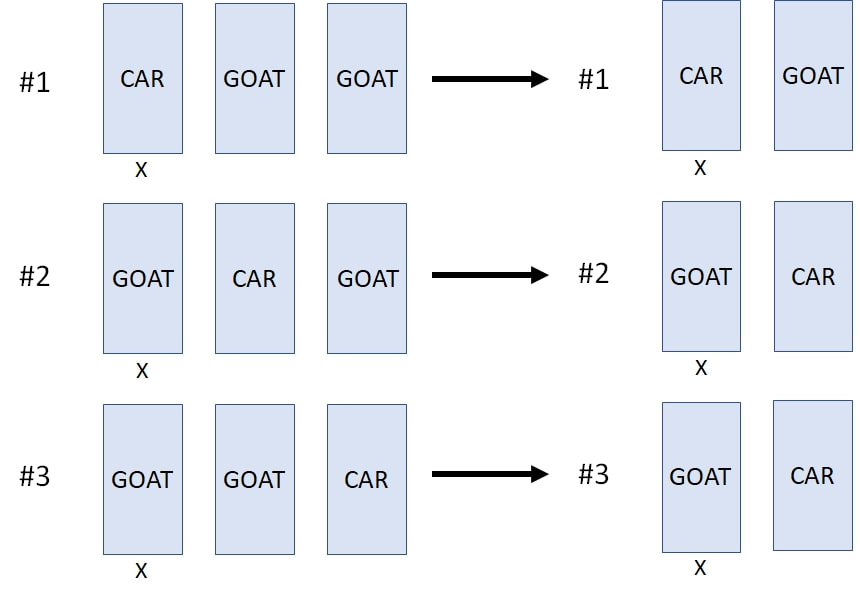
I have previously mentioned in my blog the so-called Monty Hall Puzzle (named after a Canadian-American game show host). The puzzle involves the setup of a recurring game show where you are given a choice between three doors. Behind one door is a car, and behind the other two doors there are goats. You pick one door, and the game show host proceeds to open one of the remaining two doors revealing a goat (note: he knows where the car and the goats are, and after a player makes a choice, he always opens a door revealing a goat). The game show host then asks you if you want to switch your original selection to the other door. The question is: is it to your advantage to switch your choice of doors? This puzzle was sent in 1990 to an American writer, Marylyn vos Savant, who writes a weekly column for the magazine Parade where she solves puzzles for her readers. Marylyn at the time was recognized by the folks at Guinness World Records to be the person with the highest IQ in the world before that category was eliminated from their world record groupings in 1990. Marylyn examined the puzzle and in a very matter of fact way replied that if you switch your original door choice, your chances of winning will be 2/3, but if you retain your original choice, your chances of winning will be 1/3. This ignited a huge controversy that degenerated into an insult fest. The effort to verify Marylyn’s answer ended up involving tens of thousands of people ranging from students at schools to mathematicians and statisticians from prestigious research centers in the United States. She was eventually proven to be right. Over the years I’ve brought up this puzzle several times, and the reactions that I’ve got from people when I mention the solution and try to explain it have amazed me. Their demeanor changes. Some get impatient, and some even get frankly hostile. The solution to this puzzle seems to be so counterintuitive that people feel that you are peddling nonsense to them when you reveal the correct answer, and they get mad at you. In their minds it’s as if they are holding a cup, and I come along trying to convince them that they are not holding a cup. But the solution is correct. Yes, at the end there are two doors. Yes, behind one is a goat, and behind the other is a car. But no, the probability of winning the car is not 50%. If you keep your original choice, it is 33.3% (1/3) and if you switch it is 66.6% (2/3). And in case this doesn’t amaze/confuse you enough, consider the following: Suppose that while you are in the game show pondering whether to change your original choice, a person comes in from the street. This person doesn’t know anything about what has been happening in the game show. Suppose that this person is asked to choose one of the two doors that you are looking at. What is the probability that this person would win the car if the person chooses one of these two doors at random? The amazing answer is 50%! In order to understand what’s going on, first we will start with two doors as shown in figure-1. In a random fashion, I place a car behind one of the doors and a goat behind the other, and I ask you to pick a door. Your chances of winning the car are 50%. If we repeat this trial 18 times, you will win the car 9 times out of 18 (statistically speaking). So far so good. But now suppose that I do not place the car behind the doors in a random fashion. Suppose that I always place the car behind the door on the right (figure-2). If we repeat the trial 18 times and you choose a door at random every time, you will still win the car 9 times out of 18 (50%). However, if somebody tips you off that I will always place the car behind the door on the right, and you adapt your door picking strategy to always select the door on the right (non-random choosing), you will win a car 18 times out of 18 (100%)! Of course, if you instead always pick the door on the left, you will never win a car. Please notice that in this example THERE ARE ONLY TWO DOORS, behind one is a car, and behind the other is a goat, YET if you pick the door on the left you will NEVER win a car. If you pick the door on the right, you WILL ALWAYS win a car. And if you pick at random between the two doors you will win the car HALF OF THE TIME! This illustrates the key point behind probability determination: randomness. If you know the allocation of the car to a given door is not random, you can use this information to increase your chance of winning (in the above case 100% by choosing the door on the right). Now suppose that we repeat the trial another 18 times, but I place the car behind the door on the left 6 times out of 18 (6/18 or 1/3: 33.3%), and I place the car behind the door on the right 12 times out of 18 (12/18 or 2/3: 66.6%) as shown in figure-3. If you choose a door at random, you will pick on the average the door on the left 9 times and win the car on 3 occasions, and the door on the right 9 times and win the car on 6 occasions for a grand total of 9 (6+3) times out of 18, or 50%. But again, if somebody tips you off to what I’m doing, and you always select the door on the right, you will win a car 12 times out of 18 (12/18 or 2/3: 66.6%). Of course, if you instead always pick the door on the left, you will win the car only 6 times out of 18 (6/18 or 1/3: 33.3%). Again, please notice: THERE ARE ONLY TWO DOORS, behind one a car, behind the other a goat, YET if you pick the one on the left you win a car 1/3 of the time. If you pick the one on the right, you win the car 2/3 of the time. And if you pick at random between the two doors, you win 50% of the time! At this point, even if you agree with me that lack of randomness can lead to different probabilities of winning the car when there are only two doors (depending on how you choose) you can still argue that increasing your chances of winning in the above examples depends on somebody tipping you off, in other words: cheating. But what if you could obtain this information without cheating? In the Monty Hall puzzle, there are three doors. Behind one there is a car, and behind the other two there are goats. So the three possible arrangements are #1 car-goat-goat, #2 goat-car-goat, and #3 goat-goat-car (see figure-4). IF the car is placed behind the doors at random, and you repeat the Monty Hall trial 18 times, the chance of picking the door with a car is 6 in 18 (1/3: 33.3%) whether you choose the doors at random or not. Then, after you make your choice, the game show host opens one of the two remaining doors revealing a goat and asks you if you want to change your initial pick. The key to understanding the answer to the Monty Hall puzzle is to realize that by opening the door and revealing a goat, the game show host has eliminated the element of randomness in the allocation of the car to the doors. By eliminating that extra door, the odds now favor the door opposite to the one you picked! Say that, for the sake of simplicity, out of the three doors (left, center, and right) you have chosen the door on the left (marked with an X under the door for the three possible arrangements: see below). That door will have a car behind it 1/3 of the time. But now the game show host opens one of the remaining doors revealing a goat (door crossed out). By doing this, the game show host changes the original possible three-door arrangement of #1 Car-Goat-Goat, #2 Goat-Car-Goat, and #3 Goat-Goat-Car, and converts it into a two-door arrangement: #1 Car-Goat, #2 Goat-Car, and #3 Goat-Car where your door of choice is the one on the left (marked with the X) as shown in figure-6. But notice that in the new two door scenario arrangements #2 and #3 are the same. The game show host has created a situation identical to the one depicted in the example of figure-3 where there are only two doors, and one of the doors is favored over the other when it comes to placing the car behind the doors (in this case the one opposite to the one you picked: the one on the right). Therefore, just like in the situation of figure-3, you can exploit this information by switching to the other door and increasing your probability of winning from 1/3 to 2/3. The difference, of course, is that in the example of figure-3 there was cheating involved (somebody tipped you off), whereas in the actual Monty Hall puzzle, your knowledge about how the setup came into being (the opening of the door revealing a goat) allows you to exploit it to improve your odds of winning the car. On the other hand, the person walking in from the street, who doesn’t have the information you have, will choose between the doors at random, so their chance of winning the car is 50%.
Many people automatically assume randomness when gauging the probability of an either-or event. At the end there are two doors, behind one is car and behind the other is goat, therefore thinking that there is a 50% chance of winning seems like a no-brainer. This may be why people are so confused and exasperated by the correct answer to the Monty Hall puzzle. But what my explanation illustrates is that if you can gain information about a setup and figure out that it is not random, you can use this information to increase your odds of winning by changing your picking strategy. The Monty Hall puzzle is, of course, just a puzzle, but it bears on how we conduct ourselves in the real world when making choices about either-or outcomes. Should I buy a mortgage on this house? Will the housing market go up or down? Should I buy the stock of this company? Will the stock go up or down? Should I begin looking for work? Will I get laid off or not? The probability of most real-life either-or events is determined by forces which are not random. If we understand probability and we identify these forces, we can make the odds work in our favor. Unfortunately, many people misjudge their chances or get dupped into believing false probability determinations, and they end up with, well…a goat. The Monty Hall Puzzle image by Cepheus is in the public domain, all other images belong to the author and can only be reproduced with permission.
0 Comments
5/4/2022 The Two-Way Street of Human Folly and Its Relevance for Explaining the Craziness of our TimesRead NowThe capacity of the human mind to fool itself seems to be nearly bottomless. People do not like to deal with challenges to their ideas. Thus, they can be easy prey for those who are willing to tell them what they want to hear in order to exploit them. This is the oldest trick in the book, and it has been used countless times by individuals ranging from children to emperors throughout the ages. A remarkable example of this phenomenon is presented in a documentary series entitled The Confession Killer which examines the case of Henry Lee Lucas. Lucas was a native of Virginia who had a troubled childhood marked by abuse and neglect. He ended up serving 15 years in jail for killing his mother in 1960. Upon his release, he became a drifter and was involved in a series of crimes over the years until 1983, when he was arrested in Texas and confessed to killing two women. During his arraignment, Lucas made the shocking claim that he had also killed an additional 100 women. A task force was set up to investigate these allegations, and police officers flocked to Texas from all areas of the US with unsolved crimes, and Lucas admitted to having committed them. The numbers of murders he confessed to started growing from the original 100 to 200, 300, and kept increasing up to an astounding 600! Henry Lucas understood that as long as he kept confessing to murders, he would not be sent to the state penitentiary, and instead would remain at the local jail where he developed a friendship with his jailers and had perks like his own television, cigarettes, specific choices of food, being allowed to walk around without chains, etc. He was also driven around and flown to murder sites. He developed a near rock star status with thousands of people following his every utterance, and he was even interviewed by journalist from other countries. If this phenomenon ended here, apart from the sheer volume of confessions, it would be to a certain extent unremarkable. After all, it has been known since time immemorial that people can be taken for suckers, and Henry Lee Lucas proved adept at manipulating those around him. However, what happened next is what makes this case remarkable and disturbing. Even after Lucas had been caught lying many times. Even after rock-solid evidence was produced that indicated that he could not have been the perpetrator of all those murders. And even after Lucas himself recanted his confessions and expressed his desire to stop lying, he was egged on to continue lying, in ways both subtle and overt, by the law-enforcement officers around him. Lucas had unwillingly created a Frankenstein. When police solve unsolved murders, that makes them look good. It helps their careers, provides closure for the families of the victims, and builds goodwill among their communities. Lucas was willing to take the blame, and law enforcement officers from far and wide were willing to accept Lucas’ confessions so that they could close unsolved cases and move on. Thus the pressure was placed on him to keep the confessions coming. The manipulated became the manipulators. Lucas was unconsciously or on purpose shown details about the unsolved murders which he then parroted back to the officers interviewing him. His inconsistencies and the contrarian evidence were explained away, his trustworthiness, at least for several cases, was vouched for by the officers supervising him, and some people who tried to aggressively counter what was going on received very strong pushback from law enforcement such as a district attorney who was arrested on trumped up charges and whose life and career were ruined. What Lucas and his enablers did had a deleterious impact on society. It resulted in the closing of many cases where the real murderers were still running free, and it produced a loss in confidence in law enforcement. With the advent of DNA technology, a few dozen of the cases were reexamined many years later, and the real murderers were caught. The inescapable conclusion is that human folly can be a two-way street. And this fact may be relevant to explain some of the craziness going on in our times. Why, we often ask ourselves, do many newscasters, influencers, and other media and social media personalities push blatant lies and the most absurd conspiracy theories and other debunked nonsense with regards to vaccines, the COVID-19 epidemic, global warming, the 2020 election, and other issues? Most of these people are not stupid. Many of them are highly educated and quite intelligent. Why do they do that? I believe the reason is that these people have built their brands telling their audiences what they want to hear, and just like Henry Lee Lucas, they have found out they can’t stop. Much like Lucas’ jailers who demanded that he continue confessing to an ever-growing absurd number of murders or else he would lose all his privileges, the audiences of these talking heads also demand that they keep talking to them about an ever-growing number of outlandish claims and conspiracies. And if they recant or disavow what they have said, their audiences will shun them, and they will lose their standing and their livelihood. Like Henry Lee Lucas, they find themselves feeding a beast that demands more and more of the same, regardless of the truth, the evidence, and the facts. And just like in the case of Lucas and his enablers, this is having a negative impact on society in terms of the loss of confidence in science and many of our institutions. The photograph, a mugshot of Henry Lee Lucas from the photographic records of an unknown police department, was obtained via Wikipedia. The image has been modified and is used here under the doctrine of Fair Use. |
Details
Categories
All
Archives
June 2024
|

 RSS Feed
RSS Feed